We wan to solve the following equation
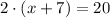
The idea to solve any equation is to find the value of the variable. To do so, we will apply mathematical operations on both sides, so we isolate the variable on one side of the equation.
First, we note that the variable is inside the parenthesis. So first, we will try to eliminate the factor that is affecting the parenthesis. To do so, we divide both sides by 2. So we get

Now, we see that the only thing that is missing, so we have the variable isolated on the left side, is to remove the 7. So, we subtract the 7 from both sides, so we get
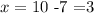
This leads to the solution x=3. Now, to check if it is correct., we take the original expression that has the variable and replace the value we found. So we have
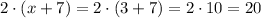
We see that the value we found those fulfills the equation, then it is a correct value.