A football player kicks a football off a tee with a speed of 16 m/s at an angle of 63°
The horizontal and vertical speed of the ball is given by

Where v = 16 m/s and θ = 63°
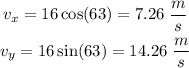
How far is the ball from the football player when it lands?
The range of the ball is given by
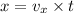
Where t is the time the ball remains in the air.
The time (t) can be found as

y = 0 when the ball is in the air.
The acceleration is due to gravity (-9.8 m/s²)

Finally, the range is
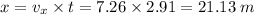
Therefore, the ball will land 21.13 meters far from the football player.
How much farther would the ball go if he kicked it with the same speed, but at a 45° angle?
We need to repeat the above calculations
The horizontal and vertical speed of the ball is given by

The time (t) is given by

Finally, the range is
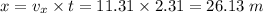
Therefore, the ball will land 26.13 meters far from the football player.
Which ball will land first: the ball kicked at 16 m/s and at a 63° angle, or one kicked at 9 m/s and at a 45° angle?
The ball kicked at 16 m/s and at a 63° angle takes 2.91 s to land.
The ball kicked at 9 m/s and at a 45° angle will take
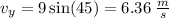

So, the ball kicked at 9 m/s and at a 45° angle takes 1.30 s to land.
Therefore, the ball kicked at 9 m/s and at a 45° angle will land first.