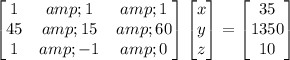Answer:
The system of matrix needed to solve the problem will be;
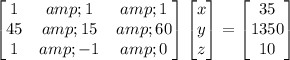
Step-by-step explanation:
Let x, y and z represent the number of cots, chairs, and tables the airport bought.
Given;
The airport bought cots, chairs, and tables totaling 35. the total number of cots, chairs and tables bought is 35. So, we have;
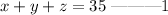
If they bought cots at $45 each, chairs for $15 each, and tables for $60 each. And they spent a total of $1350 on the furniture.
Then the total cost is the sum of the cost of each type of furniture;
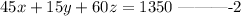
And also, The number of chairs they purchased was 10 less than the number of cots. So, we have;
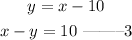
So we have the three equations needed.
We can combine the 3 equations to form a matrix.
Therefore, the system of matrix needed to solve the problem will be;