To answer this question, we need to remember the following key concept:
• A function is even if we check that,:
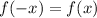
In this case, we can say that the function has been reflected in the y-axis.
• Likewise, we can say that a function is odd if we check that:
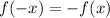
Then, we need to check these two situations with the three given functions as follows:
First case
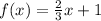
1. We need to check if the function is even:
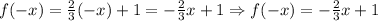
We can check that:
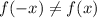
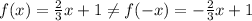
Therefore, the function is not even.
2. We need to verify if the function is odd:
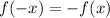
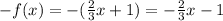
Then, we have:

Then, the function is not odd.
Therefore, the function is neither even nor odd.
Second case
We can proceed in a similar way as before:
1. We need to verify if the function is even:

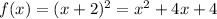

Then

Since

Thus, the function is not even.
2. Verify if the function is odd
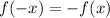
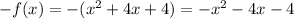
Then, we have that:
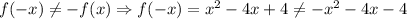
Hence, the function is not odd.
Therefore, the function is neither even nor odd.
Third Case
We have the function:

We need to remember that |x| is the function absolute value.
1. Is the function even?
Then, we have:

Then, we can see that the function is even, since:
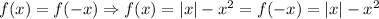
Then, the function is even.
2. Is the function odd?
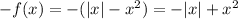
Then, we have:

Thus, the function is not odd.
Therefore, this function is even. However, it is not odd.
In summary, we have:
a. The function:
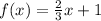
Neither even nor odd.
b. The function:
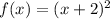
Neither even nor odd.
c. The function:

The function is even. However, it is not odd.