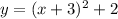When we are given a quadratic expression in the standard form:

We can complete the squares to rewrite it as:

Where:
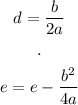
The poroblem gives us the quadratic equation:

Here, we have:
a = 1
b = 6
c = 11
Let's calculate d and e:
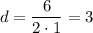
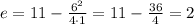
Then, we can rewrite:
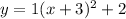
Thus, the answer is: