Starting with the equation:

Divide both sides by m, provided that m is not equal to 0:
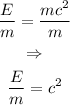
Take the square root of both sides of the equation:
![\sqrt[]{(E)/(m)}=\sqrt[]{c^2}](https://img.qammunity.org/2023/formulas/mathematics/college/awi8vg806bczrzac3chw7mc0lqby2mpuip.png)
Remember that the square root of a squared number is equal to the absolute value of that number:
![\sqrt[]{(E)/(m)}=|c|](https://img.qammunity.org/2023/formulas/mathematics/college/bh8ee2c8sqrhn4udc5a1rhjdw08cqkfhbr.png)
This equation for c has two possibilities, whether c is positive or negative:
![c=\pm\sqrt[]{(E)/(m)}](https://img.qammunity.org/2023/formulas/mathematics/college/85c7jih7qqryqj27doyhm3n7ks9b07q0bd.png)
In the context of physics, only the positive value has a physical meaning.