We are given a complex number of the form:

We are also given that arg(w) = π/4. With this information, we can calculate the value of p.
The argument of a complex number is defined as:
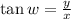
Where y and x are the imaginary and real parts (respectively) of the complex number. Applying the formula:
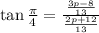
Since the tangent of π/4 is 1, the real and the imaginary parts happen to be equal, that is:
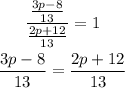
Simplifying by 13:
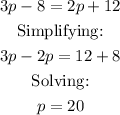
Substituting into the complex number:
w = 3 + 3i