Given the points:
(x1, y1) ==> P(-2, 3)
(x2, y2) ==> P'(-8, 12)
To find the rule that best represents the dilation, let's find the scale factor of the dilation.
To find the scale factor, divide the coordinates of the second point by the coordinates of the first point.
Thus, we have:
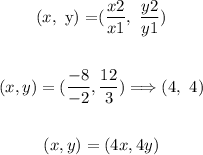
Therefore, the rule that best describes the dilation is: