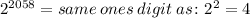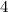
1) We need to find out a pattern for the powers that have a base 2:
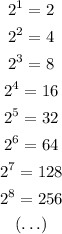
Note that from 4 to 4 powers the last digit starts to repeat itself.
2) So, let's proceed with this dividing the exponent 2058 by 4:
Now, note that the remainder is 2, therefore we can state that: