1 Answer

Nick Bauer · Answer 1 · 2023-09-11T11:32:28+0000

We will investigate how to determine the amount of money Mark started off with at the beginning off the day.

We will assume and declare a variable to Mark's bank balance at the beginning off the day:

$P\text{ = Inital balance}$

Then mark sets out for movies and gets himself snacks to enjoy along his movies. The total receipt charged for his excursion is:

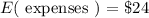
$E\text{( expenses ) = \$24}$

After his day expenses he will be left with a closing balance for the day. The closing balance of the day is expressed as:

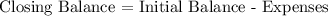
$\text{Closing Balance = Initial Balance - Expenses}$

We are given that mark was left with $12. This means:

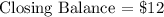
$\text{Closing Balance = \$12}$

Using the expression above we can write:

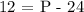
$12\text{ = P - 24}$

We will solve the above expression for initial balance ( P ) as follows:

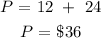
$\begin{gathered} P\text{ = 12 }+\text{ 24} \\ P\text{ = \$36} \end{gathered}$

Therefore, the answer is:

$\text{\$36}$

Please log in or register to add a comment.