Question

The beta decay of cesium-137 has a half-life of 30.0 years. How many years must pass to reduce a 25.0 mg sample of cesium-137 to 9.38 mg?

Answer 1

t = 42.4 years

Step-by-step explanation:

To find the amount of time needed for the sample to decay, you need to use the half-life equation:

`N(t) = N_0((1)/(2))^(t/h)`

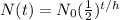
In this equation,

-----> N(t) = final mass (mg)

-----> N₀ = initial mass (mg)

-----> t = time passed (yrs)

-----> h = half-life (yrs)

You can find how much time passed by plugging the given variables into the equation and solving for "t". The final answer should have 3 sig figs like the given values.

N(t) = 9.38 mg t = ? yrs

N₀ = 25.0 mg h = 30.0 yrs

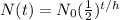`N(t) = N_0((1)/(2))^(t/h)` <----- Half-life equation

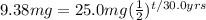`9.38mg = 25.0mg((1)/(2))^(t/30.0yrs)` <----- Insert variables

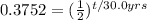`0.3752 = ((1)/(2))^(t/30.0yrs)` <----- Divide both sides by 25.0 mg

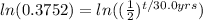`ln(0.3752) = ln(((1)/(2))^(t/30.0yrs))` <----- Take the natural log of both sides

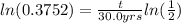`ln(0.3752) = (t)/(30.0yrs) ln((1)/(2))` <----- Rearrange the exponent

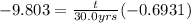`-9.803 = (t)/(30.0yrs) (-0.6931)` <----- Solve the natural logs

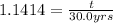`1.1414= (t)/(30.0yrs)` <----- Divide both sides by -0.6931

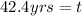`42.4 yrs= t` <----- Multiply both sides by 30.0 yrs