Question

How is f(-4) and g(-2) determined to complete the function?

Answer 1

The given functions are

`\begin{gathered} f(x)=2x^3+2 \\ g(x)=4x-3 \end{gathered}`

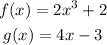
The question requires the values of

`f(-4),g(-2)`

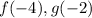
To find f(-2), substitute x=-2 into f(x)

It follows

`f(-4)=2(-4)^3+2`

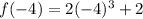
Solve for f(-4)

`\begin{gathered} f(-4)=2(-64)-2 \\ f(-4)=-126 \end{gathered}`

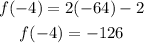
Therefore the value of f(-4)=-126.

To get g(-2) substitute x=-2 into g(x)

It follows

`g(-2)=4(-2)-3`

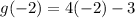
Solve for g(-2)

`\begin{gathered} g(-2)=-8+3 \\ g(-2)=-5 \end{gathered}`

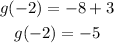
Therefore the value of g(-2)=-5.