the town's population after 17 years is 44,685
Step-by-step explanation:
decrease = rate = 3% = 0.03
t = 17 years
Present population = a = 75,000
y = population after t years
Exponential growth formula:
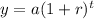
Since the poulation is decreasing, the formula becomes (exponential decay):
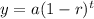
inserting into the formula:
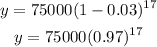
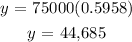
Hence, the town's population after 17 years is 44,685