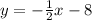Question

Hi , how do iFind the equation of the line in slope intercept and in general form going through the points (4,-10), and (-2, -7).

Answer 1

As given by the question

There are given that the two points:

`(4,\text{ -10) and (-2, -7)}`

Now,

To find the equation of line, first, find the slope of line:

Then,

From the formula of slope:

`m=(y_2-y_1)/(x_2-x_1)`

Then,

`\begin{gathered} m=(y_2-y_1)/(x_2-x_1) \\ m=\frac{-7_{}-(-10)_{}}{-2_{}-4_{}} \\ m=(-7+10)/(-6) \\ m=(3)/(-6) \\ m=-(1)/(2) \end{gathered}`

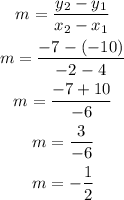
Now,

From the formula of slope-intercept form

`y=mx+b`

Then, put the value of m, y, and x to find the value of b

So

`\begin{gathered} y=mx+b \\ -10=-(1)/(2)(4)+b \\ -10=-2+b \\ -10+2=-2+b+2 \\ -8=b \end{gathered}`

Then,

Put the value of b and m into the general form of the equation

`\begin{gathered} y=mx+b \\ y=-(1)/(2)x-8 \end{gathered}`

Hence, the equation of a line is shown below:

`y=-(1)/(2)x-8`