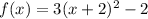x-axisThe given function is as follows, I am gonna also write the standard form of it.!
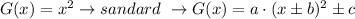
Where a, b, c are constants that adjust the apparent look and position of the function.
a changes width of the function, b translates or moves function along the x-axis, and finally, c translates or moves the function along the y-axis.
Now the function in red has the following properties:
It is shifted -2 in x and -2 in y and it is skewed or narrowed (its width is reduced )
Keeping these in mind, for x translation to go 2 units in a negative direction the 'b' has to be +2, and similarly, for y translation, the c has to be -2.
At this point keeping these values in mind, option c matches with the f(x).