Quadratic Function
The general form of a quadratic equation is:

Comparing this equation with the given function:
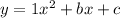
It's clear that
a=1
We don't know the values of b and c.
To find them, we use the vertex form of the quadratic equation:

Where the point (h,k) are the coordinates of the vertex.
Substituting the coordinates of the vertex (3,8):
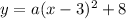
Expanding the square and substituting a=1:
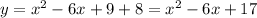
The equation is now in standard form, and we can easily identify the values of b and c:
b = -6
c = 17