Question

Consider the following function.f(x) = (x2 + 9)(6x + 2), (2, 18)==(a) Find the value of the derivative of the function at the given point.f'(2) =(b) Choose which differentiation rule(s) you used to find the derivative. (Select all that apply.)power rulequotient ruleproduct rule

Answer 1

For this problem, we are given a function and we need to determine the derivative on the given point.

The function is:

`f(x)=(x^2+9)(6x+2)`

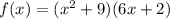
The derivative is:

`\begin{gathered} f^(\prime)(x)=(x^2+9)^(\prime)(6x+2)+(x^2+9)(6x+2)^(\prime)\\ \\ f^(\prime)(x)=2x(6x+2)+(x^2+9)(6)\\ \\ f^(\prime)(x)=12x^2+4x+6x^2+54\\ \\ f^(\prime)(x)=18x^2+4x+54 \end{gathered}`

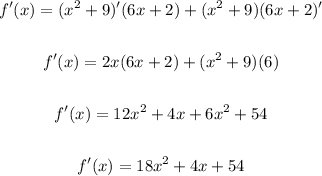
Now we need to apply the given point, which means replacing "x" with 2 and evaluating the expression.

`\begin{gathered} f^(\prime)(2)=18(2)^2+4(2)+54\\ \\ f^(\prime)(2)=18\cdot4+8+54\\ \\ f^(\prime)(2)=72+62=134 \\ \end{gathered}`

For this problem we used the following differentiation rules: power rule and product rule.