The rotation operation preserves the distance of the figure. We just change its orientation but the distance stays the same.
Rotation about 90 degrees will change the coordinates of the quadrilateral via the equation

Hence, for the vertices of the given quadrilateral on the figure, the following will be the new vertices upon rotation of the quadrilateral about 90 degrees counterclockwise.
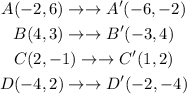
Based on the provided solution above, the answer to this problem would be yes and B'(-3,4), which is option 4.
Answer: Option 4