Here, we are given triangular prism.
To find the surface area of a triangular prism, use the formula below:
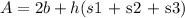
Given:
Side lengths of base, s1 = 17 yd,
s2 = 15 yd
s3 = 8 yd
height, h = 11 yd
b = area of the base
To find the area of the base which is a scalene triangle, use Heron's formula:
![\begin{gathered} A=\sqrt[]{s(s-s1)+(s-s2)+(s-s3)} \\ \\ s=(s1+s2+s3)/(2)=(17+15+8)/(2)=(40)/(2)=20 \\ \\ A=\sqrt[]{20(20-17)(20-15)(20-8)} \\ \\ A=\sqrt[]{20(3)(5)(12)}_{} \\ \\ A=\sqrt[]{3600} \\ \\ A=60\text{ square yards} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/xqs37qmklvns5q4h4o561cfc7pdl60mg7s.png)
The area of the base, b = 60 square yards.
To find the surface area of the prism, we have:


Therefore, the surface area of the triangular prism is 560 square yards
ANSWER:
560 square yards