Question

A prymaid is 1/4 inch taller than it is wide. Let h=height Let w=width. Complete the table,and graph h=w+1/4

Answer 1

Given that a pyramid is ¼ inch taller than it is wide.

This means that the height of the pyramid is ¼ larger than its width.

Thus, we have:

Width = w

Height = w + ¼

To complete the table, substitute the width given for w and evaluate.

We have:

When width, w = 1

`\begin{gathered} \text{ when width, w = 1} \\ \\ h=1+(1)/(4)=1(1)/(4)=(5)/(4) \\ \\ h=(5)/(4) \end{gathered}`
`\begin{gathered} \text{ when width, w = 2} \\ \\ h=2+(1)/(4)=2(1)/(4)=(9)/(4) \\ \\ h=(9)/(4) \end{gathered}`
`\begin{gathered} \text{when width, w = 3} \\ \\ h=3+(1)/(4)=3(1)/(4)=(13)/(4) \\ \\ h=(13)/(4) \end{gathered}`

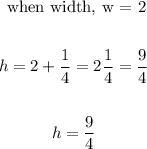
Therefore, we have the complete table below:

To plot the the graph, we have the equation of the line:

`h=w+(1)/(4)`

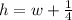
Let w represent the x-axis

Let h represent the y-axis

Mark the points on the table and plot the graph.