Area of the shaded figure is,
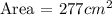
The given shaded region represents the sector of a circle . The central angle covered by the given region is,
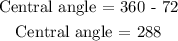
Area of a sector is given as,

The radius of the circle G is calculated as,
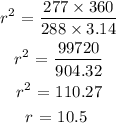
The diameter of the circle G is calculated as,
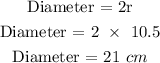
Thus the diameter of the given circle is 21 cm.