Given:
Ethan is 23 years older than Evan
So, let Ethan is (x) years old
And Evan is (y) years old
So, the difference between them is 23 years old
so, x - y = 23
And the sum of Ethan and Evan's age is 43
so, x + y = 43
So, we have the following system of equations:
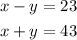
add the equations to eliminate (y):

Now, substitute with (x) into the second equation to find (y):
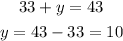
So, the answer will be:
Ethan's age = x = 33
Evan's age = y = 10