Question

Given lines l, m and n are all parallel and cut by two transversal lines, find the value of 2. 2 12 6. т х 33 n

Answer 1

66

Step-by-step explanation:

By the Thales theorem, if we have the following figure:

Then, the following equation applies:

`(a)/(c)=(b)/(d)`

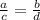
So, in this case, we can formulate the following equation:

`(6)/(12)=(33)/(x)`

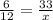
Then, solving for x, we get:

`\begin{gathered} 6\cdot x=33\cdot12 \\ 6x=396 \\ (6x)/(6)=(396)/(6) \\ x=66 \end{gathered}`

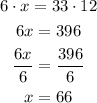
Therefore, the value of x is 66.