1) To get the vertex of the function, the formulae is given as:

This gives the x coordinate of the vertex. Where a and b are the coefficients of the 1st and 2nd terms respectiely.
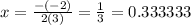
To get the y coordinate, we substitute this x value into the original equation.
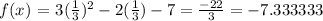
The coordinates of the the vertex (0.33, -7.33)
2) The graph opens upwards. Because the coefficient of the 2nd power of x is a negative number.
3) The -7 tells us that the graph cuts the vertical axis at -7.