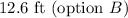
Step-by-step explanation:
The ladder leaning on the house formed a right angled triangle
Using an illustration from the diagram given:
To get the height the house makes with the ladder, we will apply pythagoras' theorem:
Hypotenuse² = opposite² + adjacent²

![\begin{gathered} \text{square root both sides:} \\ \sqrt[]{160}\text{ = }\sqrt[]{opposite^2} \\ \text{opposite = }12.649 \\ \\ \text{To the nearest tenth, the height of the house betw}een\text{ the ladder and base of the house is 12.6 ft (option B)} \end{gathered}]()