Question

Write the first five terms of the geometric sequence in which a = 64 and the common ratio is 5/4.

Answer 1

A. 64,80,100,125, 625/4

Explanation:

Given a geometric sequence with the following:

• First term, a1 = 64

,

• Common ratio, r = 5/4

We want to determine the first five terms of the sequence.

The nth term of a geometric sequence is calculated using the formula below:

`T_n=a_1(r^(n-1))`

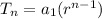
Thus, the 2nd to 5th terms are calculated below.

`\begin{gathered} T_2=64((5)/(4))^(2-1)=64((5)/(4))^1=80 \\ T_3=64((5)/(4))^(3-1)=64((5)/(4))^2=100 \\ T_4=64((5)/(4))^(4-1)=64((5)/(4))^3=125 \\ T_5=64((5)/(4))^(5-1)=64((5)/(4))^4=(625)/(4) \end{gathered}`

Therefore, the first five terms of the geometric sequence are:

`64,80,100,125\text{ and }(625)/(4)`

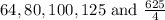
Option A is correct.