Step-by-step explanation:
We are given the graph of a function

The graph is:
And we need to find the corresponding graph for the function
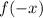
According to the reflection rules for functions:

This means that the graph of f(-x) will be a reflection across the y-axis, like a mirror, of the function f(x).
That reflection is presented in the following graph:
Which is option B.
Answer:
Option B.