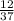Question

In ABCD, the measure of ZD=90°, BD = 12, DC = 35, and CB = 37. What ratiorepresents the sine of ZC?

Answer 1

Draw a diagram to visualize the triangle:

The sine of an angle in a right triangle is defined as the quotient between the side opposite to that angle and the hypotenuse.

The side opposite to the angle C is BD, and the hypotenuse of the triangle is CB. Then:

`\sin (\angle C)=(BD)/(CB)`

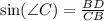
Substitute the lengths of BD and CB to find the ratio that represents the sine of the angle C in this case:

`\sin (\angle C)=(12)/(37)`

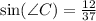
Therefore, the answer is:

`(12)/(37)`