Parallel lines have the same slope. To determine the slope of the given line, we can choose 2 points in it, (x₁, y₁) and (x₂, y₂). Using these points, we can determine the slope by:

From the graph, we can pick any points in the line, for example, we can see that the line passes through points (0, -7) and (-3, -5). Using these, we have:
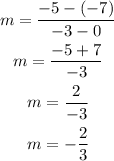
Thus, the slope of the original line is -2/3. Since the slopes of parallel lines are the same, the slopeof the parallel line is also -2/3.
One simple way of drawing a parallel line is to choose two points on the original line and adding a any number to both y-coordinates of the points.
For example, for the choseon points