Question

In the diagram below of triangle UVW, X is a midpoint of UV and Y is a midpoint of VW. If mZY XV = 3x + 48, and mZWUX = x + 62, what is the measure of ZWUX? V X Y U W

Answer 1

Given:

m∠YXV = 3x + 48

m∠WUX = x + 62

Using the corresponding angle theorem, since X is the midpoint of UV and Y is the midpoint VW, m∠YXV is congruent to m∠WUX.

Thus, we have:

m∠YXV = m∠WUX

3x + 48 = x + 62

Solve for x.

Subtract x from both sides:

3x - x + 48 = x - x + 62

2x + 48 = 62

Subtract 48 from both sides:

2x + 48 - 48 = 62 - 48

2x = 14

Divide both sides by 2:

`\begin{gathered} (2x)/(2)=(14)/(2) \\ \\ x=7 \end{gathered}`

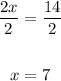
To find m∠WUX, where x = 7, we have:

m∠WUX = x + 62

= 7 + 62

= 69º

ANSWER:

m∠WUX = 69º