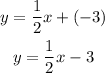Question

Please help me find the linear equation of question 9

Answer 1

Equation of a line in slope-intercept form

y = mx + b

where m is the slope and b is the y-intercept

From the graph, we can see that the line intercepts the y-axis at the point (0,-3), then b = -3.

The slope of the line that passes through the points (x1, y1) and (x2, y2) is computed as follows:

`m=(y_2-y_1)/(x_2-x_1)`

In this case, the line passes through the points (0, -3) and (2, -2), then its slope is:

`m=(-2-(-3))/(2-0)=(-2+3)/(2)=(1)/(2)`

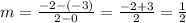
Substituting with m = 1/2 and b = -3 into the equation, we get:

`\begin{gathered} y=(1)/(2)x+(-3) \\ y=(1)/(2)x-3 \end{gathered}`