Given that
y = 1/4x + 4
x + y = -1
For the first equation,
Let us find the x intercept by making x equals to 0
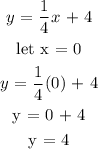
To find x, let y = 0

Therefore, we have (0, 4) and (-16, 0)
For the second equation
x + y = -1
To find the x - intercept, make x = 0
0 + y = -1
y = -1
(0, -1)
To find x, let y = 0
x + y = -1
x + 0 = -1
x = -1
(-1, 0)
Therefore, the two points are (-1, 0) and (0, -1)
The above points can now be graph
The diagram above is just an illustration on how to graph the given points
From the numbered graph, their point of intersection is (-4, 3)
x = -4 and y = 3