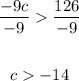Answer:
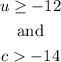
Step-by-step explanation:
Given the inequalities:
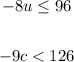
To solve these, first of all note that: Dividing both sides of an inequality by a negative number changes the inequality sign to the opposite.
For

Divide both sides of the inequality by -8
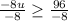
Therefore
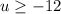
For

Divide both sides of the inequality by -9