To answer this question, we need to take into account that:
1. A polynomial is a finite sum of terms.
2. All the variables have a whole number as an exponent.
3. None of the variables appear in a denominator.
Having this information, we can say that:
First case:
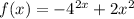
We can see that this IS NOT a polynomial, in the sense that the term -4^(2x) is an exponential function.
Second Case:
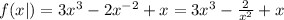
For this case, we see that in the second term, the variable appears in the denominator. Therefore, this is NOT a polynomial function.
Third Case:
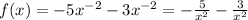
This case is similar to the previous one. Then, this is NOT a polynomial function.
Fourth Case:
![f(x)=x^2+\sqrt[]{3}x-7](https://img.qammunity.org/2023/formulas/mathematics/college/bwyaibaupp678j6oycy80bppo47gz9db9z.png)
In this case, we can see that the exponents in the variables are whole numbers (2 and 1). None of the variables appear in the denominator, and it is a finite number of terms. Therefore, this IS a polynomial function (even if it has radical 3).
Hence, the only function that is a polynomial is option D.