Answer:
The vertex is (-4, 3)
The axis of symmetry is at x = -4
The graph is shown in the explanation below
Step-by-step explanation:
Given the function:
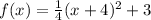
The general form of a parabola is:

The vertex is (h, k)
Comparing to the give parabola, this is (-4, 3)
The axis of symmetry is at x = h
x = -4
The graph is shown below: