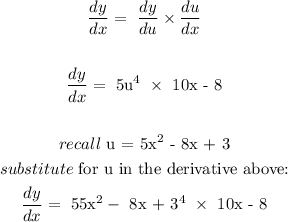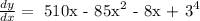Answer:
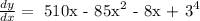
See other answers for the blanks below
Step-by-step explanation:
Given:
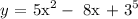
To find:
derivative of the function = dy/dx
To determine the derivative, we will follow the steps provided in the question

b) Next is to find the derivative of the outside and insides:

c) We will use the chain rule formula to get the derivative: