Question

I Need help with this problem. Quick answer is OK

Answer 1

Given:

an equation is given as below

`-7\cdot20^(x-4)=-49`

Find:

we have to solve the equation.

Step-by-step explanation:

Divide both sides of the equation by -7, we get

`20^(x-4)=7`

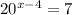
Now, by taking log on both sides, we have

`\begin{gathered} log20^(x-4)=log7 \\ (x-4)log20=log7 \\ (x-4)(1.3010)=0.8451 \\ x-4=(0.8451)/(1.3010) \\ x-4=0.6496 \\ x=0.6496+4 \\ x=4.6496 \end{gathered}`

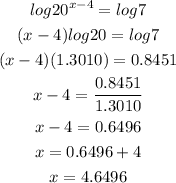
Therefore, the value of x = 4.6496

So, correct option is D, i.e. x = 4.6496