Question

Water has a pressure of 3.00 x 105 Pa and a speed of 1.00 m/s as it flows through a horizontal pipe. At a certain point, the pipe narrows to one-fourth its original diameter. (Remember the radius is half of the diameter, so its radius will also be one-fourth its original size.) What is the speed of the water flow in the narrow section of the pipe?. a.. 16.0 m/s. c.. 16.2 m/s. b.. 12.6 m/s. d.. 22.6 m/sI don’t understand where 16.0 is coming from. Can you help me? When I tried, I got 4.

Answer 1

We will have the following:

We will have that the flowrate of the water will be equal to the speed of flow multiplied by the area of flow, that is:

`F=v\cdot A=v\cdot\pi r^2`

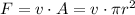
In this particular case the flowrate remains constant under a given pressise, so:

`v_1\cdot r^2_1=v_2\cdot r^2_2`

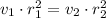
That is:

`(1m/s)(r^2_1)=v_2\cdot((r_1)/(4))^2`

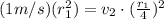
From this we can see that the value of the speed in the narrower diamter is expected to increase, So:

`(1m/s)\cdot r^2_1=v_2\cdot(r^2_1)/(16)\Rightarrow1m/s=v_2\cdot(1)/(16)`
`\Rightarrow v_2=16m/s`

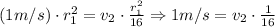
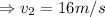
So, the velocity will be 16 m/s.