Question

The data set represents the ages of players in a chess club.27, 34, 38, 16, 22, 45, 54, 60What is the mean absolute deviation of the data set? 12.2515.42537

Answer 1

The mean absolute deviation of a data set is the average absolute difference between each data point and the mean.

First, find the mean of the data set.

There are 8 players in the chess club. Then, the average age of the players is:

`(27+34+38+16+22+45+54+60)/(8)=37`

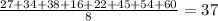
Take the absolute value of the difference between the age of each participant and the average age of 37 years:

`\begin{gathered} |27-37|=10 \\ |34-37|=3 \\ |38-37|=1 \\ |16-37|=21 \\ |22-37|=15 \\ |45-37|=8 \\ |54-37|=17 \\ |60-37|=23 \end{gathered}`

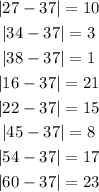
Next, find the mean of the absolute differences to find the mean absolute deviation:

`(10+3+1+21+15+8+17+23)/(8)=12.25`

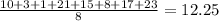
Therefore, the mean absolute deviation of the data set is 12.25.