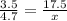Question

James has two proportional pictures. The first picture has a length of 3.5 in and a width of 4.7 in. The second picture has a length of 17.5 in. He sets up the proportion below to begin trying to find the area. Do you agree? Why or why not?

Answer 1

Since the two pictures are proportional, there should be the same constant of proportionality between their width and length.

In this case Jame has this set up for the proportion:

`(3.5)/(4.7)=(x)/(17.5)`

he has in the first fraction the length of the first picture 3.5 (the numerator) and the width 4.7 of the first picture in the denominator.

For this to be proportional, in the second part of the equation (the right part) there should also be a length in the numerator and a width in the denominator.

We can see that this is not true, because 17.5 is a length, and we should have a width in the denominator, we can see this better in the following image:

In the place of 17.5 there should be the width "x" .

So I don't agree because the right set up is the following:

`(3.5)/(4.7)=(17.5)/(x)`