Step-by-step explanation
Given the exponential function:
45(1.085)^t
We have that 45=a is the initial value and 1.085 is the growth rate,
1.085 - 1 = 0.085* 100 = 8.5 % (This is the growth rate)
Let's compute the function with two values of t, as for instance, t=0 and t=1:
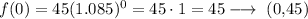
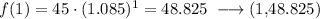
Now, the function in the form y = ae^kt will be as follows:
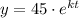
Substituting t by 1:
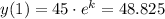
Dividing both sides by 45:
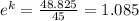
Applying ln to both sides:
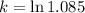
Computing the argument:

The expression will be as follows:
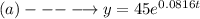
As this is a growing function, the rates are positive.
The annual growth rate is 8.5% and It's was calculated above.
Now, we need to compute the continuous rate because It's given by the value of k:
k = 0.0816 --> Multiplying by 100 --> 0.0816 * 100 = 8.16%
The continuous growth rate is 8.16%