We have the following quadratic Function in a graph:
Now, we solve one aspect at a time:
Zeros
Let's remember that the zeros or roots of the quadratic function are those values of x for which the expression is 0. Graphically, the roots correspond to the abscissae of the points where the parabola intersects the x-axis.
By looking at the graph we can identify these, which in this case it is:
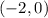
Axis of symmetry
Recall that the graph of a quadratic function is a parabola. The axis of symmetry of a parabola is a vertical line that divides the parabola into two congruent halves. The axis of symmetry always passes through the vertex of the parabola.
By looking at the graph we can identify this, which in this case it is:

Max or min
In mathematical analysis, the maxima and minima of a function, known collectively as extrema (the plural of extremum), are the largest and smallest value of the function, either within a given range or on the entire domain.
By looking at the graph we can identify this, which in this case it is:

Vertex
Remember that the vertex of a quadratic equation or parabola is the highest or lowest point of the graph corresponding to that function. The vertex lies in the plane of symmetry of the parabola; whatever happens to the left of this point will be an exact reflection of what happens to the right.
By looking at the graph we can identify this, which in this case it is: