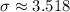Knowing the information given in the exercise:
- Anita's reports that 45% of its customers order their food to go.
- A random sample of 50 individual customers is taken.
(a) You need to find the Mean:
1. You know that if you have a Binomial Distribution, then, you can approximate the Mean to a Normal Distribution of:

Where μ is the Mean, "n" is the number of customers of the sample, and "p" is the proportion of customers that order their food to go.
2. In this case:

Then, substituting values and evaluating, you get:
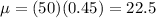
(b) You need to use the following formula to find the Standard Deviation:
![\sigma=\sqrt[]{np(1-p)}](https://img.qammunity.org/2023/formulas/mathematics/high-school/x64mzlr9vbbqzix697083fm9r7c6mdgyjs.png)
Where σ is the Standard Deviation, "n" is the number of customers of the sample, and "p" is the proportion of customers that order their food to go.
Knowing all the values, you can substitute them into the formula and evaluate:
![\begin{gathered} \sigma=\sqrt[]{(50)(0.45)(1-0.45)} \\ \\ \sigma=\sqrt[]{(22.5)(0.55)} \\ \\ \sigma\approx3.518 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/apcvfzt8xz2qs3lobb5nq9lsyxthjaxfrw.png)
Hence, the answers are:
(a)
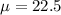
(b)