Given
The degree measure of one of two complementary angles is 9 more than twice that of the other.
To find:
What is one of the degree measures of the angles?
Step-by-step explanation:
It is given that,
The degree measure of one of two complementary angles is 9 more than twice that of the other.
Let x be the degree measure of one of the two complementary angles.
Then,
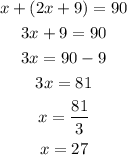
Hence, one of the degree measures of the angles is 27 degrees.