The general exponential growth model is defined as
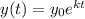
Here ley y be the population of a bacteria at ttime in hours, k is the growth parameter, t is the time, y0 is the initial population of the bacteria.
Accoridng to this problem the initial population is 2300.
So,
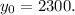
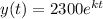
It is given that after 2 hours, the population of the bacetria is 2450.
That is, at time t=2,y=2450.
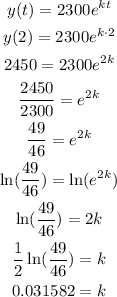
Now to get the percentage multiply the obatined k value by 100.
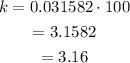
So, the required growth rate is k=3.16%.