Question

2. Of 500 newly admitted college students 220 are enrolled in Intermediate Algebra 320are enrolled in English 1 and 125 are enrolled in bothPorta: Complete the following Venn Diagram with the number of elements in eachsectionA = Intermediate AlgebraB = English 1APort & How many elements are in the subset (ans)Port How many college students are in aPort De How many college students are in the subset -(au)

Answer 1

Part A.

It is important to know that the 125 students enrolled in both refer to the intersection. So, the complete diagram would be

Also, observe that there are 85 students outside the sets, that numbers refer to the students who are not enrolled in Algebra nor English 1. We got this number by doing the following process

`\begin{gathered} 320+220-125=415 \\ 500-415=85 \end{gathered}`

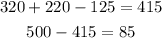
Part B.

The elements of the intersection are 125

`P(A\cap B)=125`

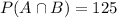
Remember that the intersection refers to the elements that are present in both sets at the same time.

Part C.

The number of college students in A is 220 because.

Part D.

At last, the subset

`\approx(A\cup B)`

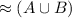
Refers to the students who are not enrolled in any of the given subjects, which are 85.