Question

Intelligent quotient scores are often reported to be normally distributed with(see picture)A sample of 41 people is taken randomly. What is the probability of a random person on the street having an IQ score of less than 99? Round your answer to four decimal places if necessary

Answer 1

Step-by-step explanation

The z-score of a value x that is part of a normally distributed population is given by the following equation:

`z=(x-\mu)/(\sigma)`

Where μ is the mean of the population and σ is the standard deviation.

The probability of finding an element lower than x is given by the area under the normal curve (centered around 0 and with a standard deviation of 1) at the left of the z-score associated with x.

This basically means that we must find the z-score for an IQ of 99 and look for it in a z-score table showing the area at the left of it. This area will be the probability that we are looking for.

Then we find the z-score of x=99 with a mean of 100 and a standard deviation of 15:

`z=(99-100)/(15)=-0.07`

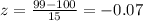
So we look for z=-0.07 in a z-score table:

As you can see the area under the curve at the left of z=-0.07 is 0.4721 and this is the probability that we were looking for.

Answer

Then the answer is 0.4721