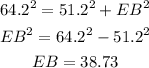Remember that
A perpendicular bisector divides a segment into two equal parts
so
AD=DB=61.7
In the right triangle DYB
Apply the Pythagorean Theorem
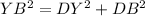
where
YB=64.2
DB=61.7
substitute given values
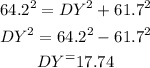
Find out the value of EB
In the right triangle YEB
Apply the Pythagorean Theorem
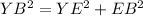
where
YB=64.2
YE=51.2
substitute given values