Given
One of the zeros of 14² − 42² − 9 is the negative of the other.
To find the value of k.
Step-by-step explanation:
It is given that,
One of the zeros of 14² − 42² − 9 is the negative of the other.
That implies,
Let α, -α be the zeros of the polynomial f(x) = 14² − 42² − 9.
Then,
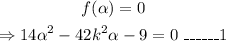
Also,
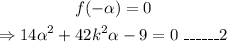
Subtracting (1) and (2) implies,
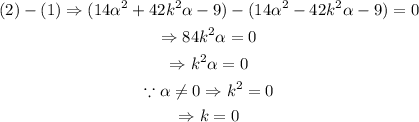
Hence, the value of k is 0.