The given equation is:
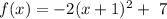
The equation of a parabola can be expressed in vertex form as:
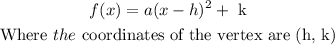
Comparing this formula with then function given:
a = -2
h = -1
k = 7
The vertex = (h, k)
Vertex = ( -1, 7)
Note that:
If in a parabola, the x is squared, the parabola either opens up or down
If the multiplier, a, is positive, then it is certain that the parabola opens up
If the multiplier is negative, then the parabola opens down.
In the equation of the parabola given, that is, f(x)=-2(x+1)²+7:
a = -2 (negative), then the parabola opens down.